|
1 |
A fair die is rolled. The probability that 1 occurs at the even number of trail is a) 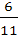 b)  c) 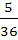 d) 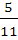
A fair die is rolled. The probability that 1 occurs at the even number of trail is a) 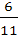 b)  c) 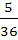 d) 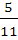
|
IIT 2005 |
05:00 min
|
|
2 |
Fill in the blank If the quadratic equation
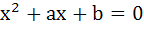 and and 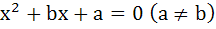 have a common root then the numerical value of a + b is ………… have a common root then the numerical value of a + b is …………
Fill in the blank If the quadratic equation
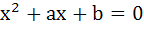 and and 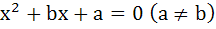 have a common root then the numerical value of a + b is ………… have a common root then the numerical value of a + b is …………
|
IIT 1986 |
01:36 min
|
|
3 |
Show that 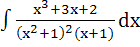 = =
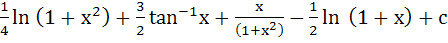
Show that 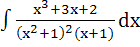 = =
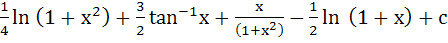
|
IIT 1999 |
09:29 min
|
|
4 |
Let a, b, c be distinct non-negative numbers. If the vectors 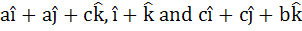 lie in a plane then c is lie in a plane then c is a) Arithmetic mean of a and b b) Geometric mean of a and b c) Harmonic mean of a and b d) Equal to zero
Let a, b, c be distinct non-negative numbers. If the vectors 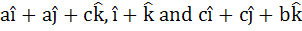 lie in a plane then c is lie in a plane then c is a) Arithmetic mean of a and b b) Geometric mean of a and b c) Harmonic mean of a and b d) Equal to zero
|
IIT 1993 |
01:42 min
|
|
5 |
(One or more correct answers)
For two given events A and B, P (A ∩ B) is a) Not less than P (A) + P (B) − 1 b) Not greater than P (A) + P (B) c) Equal to P (A) + P (B) − P (A ∪ B) d) Equal to P (A) + P (B) + P (A ∪ B)
(One or more correct answers)
For two given events A and B, P (A ∩ B) is a) Not less than P (A) + P (B) − 1 b) Not greater than P (A) + P (B) c) Equal to P (A) + P (B) − P (A ∪ B) d) Equal to P (A) + P (B) + P (A ∪ B)
|
IIT 1988 |
01:39 min
|
|
6 |
Fill in the blank The sum of the real roots of the equation
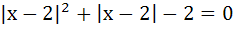 is ……….. is ………..
Fill in the blank The sum of the real roots of the equation
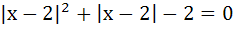 is ……….. is ………..
|
IIT 1997 |
03:01 min
|
|
7 |
If 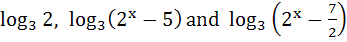 are in Arithmetic Progression, determine the value of x. are in Arithmetic Progression, determine the value of x.
If 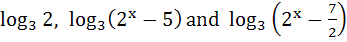 are in Arithmetic Progression, determine the value of x. are in Arithmetic Progression, determine the value of x.
|
IIT 1990 |
02:49 min
|
|
8 |
Let F(x) be an indefinite integral of sin2x
Statement 1: The function F(x) satisfies F(x + π) = F(x) for all real x because
Statement 2: sin2(x + π) = sin2x for all real x Then which one of the following statements is true? a) Statement 1 and 2 are true statements and Statement 2 is a correct explanation of Statement 1 b) Statement 1 and 2 are true statements and statement 2 is not a correct explanation of statement 1 c) Statement 1 is true, Statement 2 is false d) Statement 1 is false, Statement 2 is true
Let F(x) be an indefinite integral of sin2x
Statement 1: The function F(x) satisfies F(x + π) = F(x) for all real x because
Statement 2: sin2(x + π) = sin2x for all real x Then which one of the following statements is true? a) Statement 1 and 2 are true statements and Statement 2 is a correct explanation of Statement 1 b) Statement 1 and 2 are true statements and statement 2 is not a correct explanation of statement 1 c) Statement 1 is true, Statement 2 is false d) Statement 1 is false, Statement 2 is true
|
IIT 2007 |
02:04 min
|
|
9 |
Let 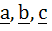 are non–coplanar unit vectors such that are non–coplanar unit vectors such that 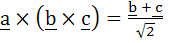 then the angle between a and b is then the angle between a and b is a) 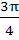 b) 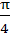 c)  d) π
Let 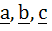 are non–coplanar unit vectors such that are non–coplanar unit vectors such that 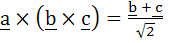 then the angle between a and b is then the angle between a and b is a) 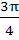 b) 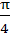 c)  d) π
|
IIT 1995 |
02:20 min
|
|
10 |
The number 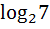 is is a) an integer b) a rational number c) an irrational number d) a prime number
The number 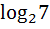 is is a) an integer b) a rational number c) an irrational number d) a prime number
|
IIT 1992 |
00:47 min
|
|
11 |
The fourth power of the common difference of an arithmetic progression with integer entries is added to the product of four consecutive terms of it, prove that the resulting sum is square of an integer.
The fourth power of the common difference of an arithmetic progression with integer entries is added to the product of four consecutive terms of it, prove that the resulting sum is square of an integer.
|
IIT 2000 |
02:57 min
|
|
12 |
If a 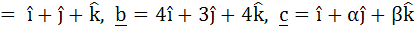 are linearly dependent and |c| are linearly dependent and |c| then then a)  b) 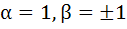 c) 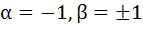 d) 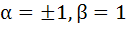
If a 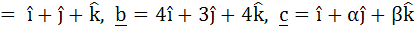 are linearly dependent and |c| are linearly dependent and |c| then then a)  b) 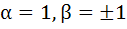 c) 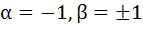 d) 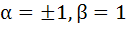
|
IIT 1998 |
04:11 min
|
|
13 |
Six boys and six girls sit in a row at random. Find the probability that the girls and the boys sit alternately.
Six boys and six girls sit in a row at random. Find the probability that the girls and the boys sit alternately.
|
IIT 1978 |
05:30 min
|
|
14 |
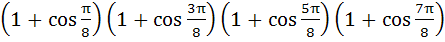 is equal to is equal to
a)  b) 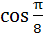 c)  d) 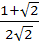
|
IIT 1984 |
03:04 min
|
|
15 |
If a, b, c are positive real numbers then prove that
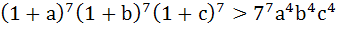
If a, b, c are positive real numbers then prove that
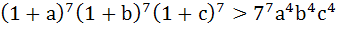
|
IIT 2004 |
02:42 min
|
|
16 |
Let f(x) be a quadratic expression which is positive for all values of x. If g(x) =  then for any real x then for any real x a) g (x) < 0 b) g (x) > 0 c) g (x) = 0 d) g (x) ≥ 0
Let f(x) be a quadratic expression which is positive for all values of x. If g(x) =  then for any real x then for any real x a) g (x) < 0 b) g (x) > 0 c) g (x) = 0 d) g (x) ≥ 0
|
IIT 1990 |
02:54 min
|
|
17 |
If  and and 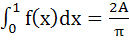 , then constants A and B are , then constants A and B are a) 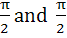 b) 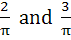 c) 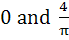 d) 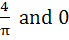
If  and and 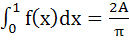 , then constants A and B are , then constants A and B are a) 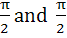 b) 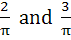 c) 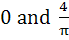 d) 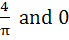
|
IIT 1995 |
02:11 min
|
|
18 |
If the vectors  form sides BC, CA and AB respectively of a triangle ABC then form sides BC, CA and AB respectively of a triangle ABC then a) 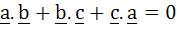 b) 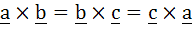 c)  d) 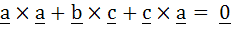
If the vectors  form sides BC, CA and AB respectively of a triangle ABC then form sides BC, CA and AB respectively of a triangle ABC then a) 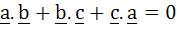 b) 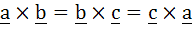 c)  d) 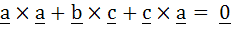
|
IIT 2000 |
02:48 min
|
|
19 |
Cards are drawn one by one at random from a well shuffled pack of 52 playing cards until 2 aces are drawn for the first time. If N is the number of cards required to be drawn show that
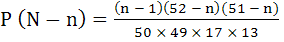 where 2 < n ≤ 50 where 2 < n ≤ 50
Cards are drawn one by one at random from a well shuffled pack of 52 playing cards until 2 aces are drawn for the first time. If N is the number of cards required to be drawn show that
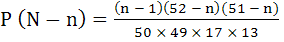 where 2 < n ≤ 50 where 2 < n ≤ 50
|
IIT 1983 |
07:44 min
|
|
20 |
If the lengths of the sides of a triangle are 3, 5, 7 then the largest angle of the triangle is a)  b) 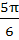 c)  d) 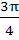
If the lengths of the sides of a triangle are 3, 5, 7 then the largest angle of the triangle is a)  b) 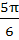 c)  d) 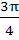
|
IIT 1994 |
01:44 min
|
|
21 |
If y = y (x) and it follows the relation xcosy + ycosx = π then 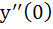 is is a) – 1 b) π c) – π d) 1
If y = y (x) and it follows the relation xcosy + ycosx = π then 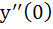 is is a) – 1 b) π c) – π d) 1
|
IIT 2005 |
03:40 min
|
|
22 |
Let f be a positive function. Let
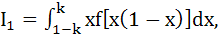
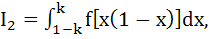 where where
2k – 1 > 0 then  is is a) 2 b) k c)  d) 1
Let f be a positive function. Let
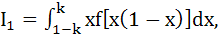
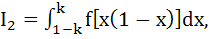 where where
2k – 1 > 0 then  is is a) 2 b) k c)  d) 1
|
IIT 1997 |
02:23 min
|
|
23 |
Let
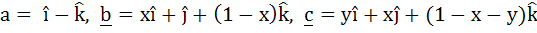 , ,
then 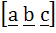 depends on depends on a) Only x b) Only y c) Neither x nor y d) Both x and y
Let
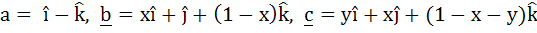 , ,
then 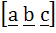 depends on depends on a) Only x b) Only y c) Neither x nor y d) Both x and y
|
IIT 2001 |
01:20 min
|
|
24 |
In a multiple choice question there are four alternative answers out of which one or more is correct. A candidate will get full marks in the question only if he ticks the correct answers. If he is allowed up to three chances to answer the question, find the probability that he will get marks in the question?
In a multiple choice question there are four alternative answers out of which one or more is correct. A candidate will get full marks in the question only if he ticks the correct answers. If he is allowed up to three chances to answer the question, find the probability that he will get marks in the question?
|
IIT 1985 |
05:36 min
|
|
25 |
Let the Harmonic Mean and Geometric Mean of two positive numbers be in the ratio of 4:5. Then the two numbers are in the ratio . . . . .
Let the Harmonic Mean and Geometric Mean of two positive numbers be in the ratio of 4:5. Then the two numbers are in the ratio . . . . .
|
IIT 1992 |
02:26 min
|