|
126 |
If the algebraic sum of the perpendicular distance from the point
(2, 0), (0, 2) and (1, 1) to a variable straight line be zero then the line passes through a fixed point whose coordinates are
If the algebraic sum of the perpendicular distance from the point
(2, 0), (0, 2) and (1, 1) to a variable straight line be zero then the line passes through a fixed point whose coordinates are
|
IIT 1991 |
03:15 min
|
|
127 |
The general solution of
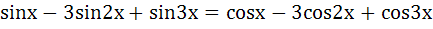 is is a) 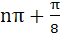 b) 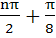 c) 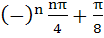 d) 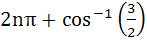
The general solution of
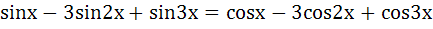 is is a) 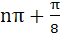 b) 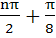 c) 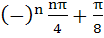 d) 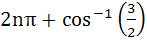
|
IIT 1989 |
03:28 min
|
|
128 |
The function f(x) = 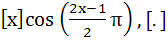 denotes the greatest integer function is discontinuous at denotes the greatest integer function is discontinuous at a) All x b) All integer points c) No x d) x which is not an integer
The function f(x) = 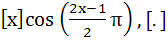 denotes the greatest integer function is discontinuous at denotes the greatest integer function is discontinuous at a) All x b) All integer points c) No x d) x which is not an integer
|
IIT 1993 |
03:16 min
|
|
129 |
If f (x) and g (x) are continuous functions on (0, a) satisfying f (x) = f (a – x) and g (x) + g (a – x) = 2 then show that 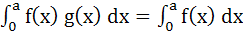
If f (x) and g (x) are continuous functions on (0, a) satisfying f (x) = f (a – x) and g (x) + g (a – x) = 2 then show that 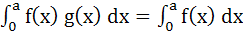
|
IIT 1989 |
02:36 min
|
|
130 |
The equation of the circles through (1, 1) and the point of intersection of
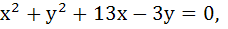
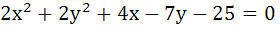 is is a)  b)  c) 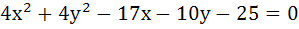 d) None of these
The equation of the circles through (1, 1) and the point of intersection of
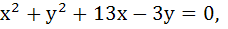
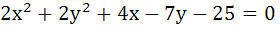 is is a)  b)  c) 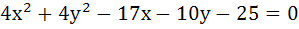 d) None of these
|
IIT 1983 |
02:31 min
|
|
131 |
The general value of θ satisfying the equation
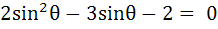 is is a) 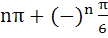 b)  c) 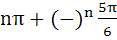 d) 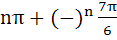
The general value of θ satisfying the equation
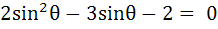 is is a) 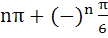 b)  c) 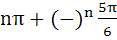 d) 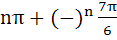
|
IIT 1995 |
01:18 min
|
|
132 |
A cubic f (x) vanishes at x = −2 and has a relative minimum/maximum at x = −1 and 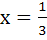 . If . If 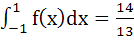 , find the cube f (x). , find the cube f (x). a) x3 + x2 + x + 1 b) x3 + x2 − x + 1 c) x3 − x2 + x + 2 d) x3 + x2 − x + 2
A cubic f (x) vanishes at x = −2 and has a relative minimum/maximum at x = −1 and 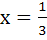 . If . If 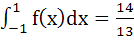 , find the cube f (x). , find the cube f (x). a) x3 + x2 + x + 1 b) x3 + x2 − x + 1 c) x3 − x2 + x + 2 d) x3 + x2 − x + 2
|
IIT 1992 |
05:24 min
|
|
133 |
If a circle passes through the points (a, b) and cuts the circle 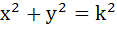 orthogonally, then the equation of the locus of its centre is orthogonally, then the equation of the locus of its centre is a)  b) 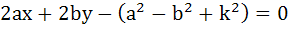 c) 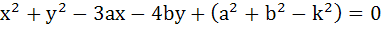 d) 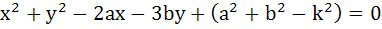
If a circle passes through the points (a, b) and cuts the circle 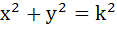 orthogonally, then the equation of the locus of its centre is orthogonally, then the equation of the locus of its centre is a)  b) 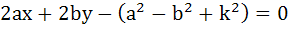 c) 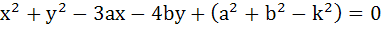 d) 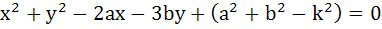
|
IIT 1988 |
04:03 min
|
|
134 |
In ΔPQR, angle R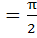 . If tan . If tan  and tan and tan  are roots of the equation are roots of the equation 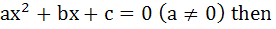 a)  b) 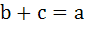 c) 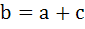 d) 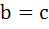
|
IIT 1999 |
02:23 min
|
|
135 |
Prove that 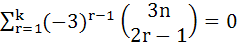
where  and n is an even integer. and n is an even integer.
Prove that 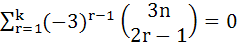
where  and n is an even integer. and n is an even integer.
|
IIT 1993 |
09:38 min
|
|
136 |
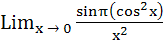 equals equals
a) – π b) π c)  d) 1
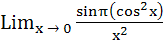 equals equals
a) – π b) π c)  d) 1
|
IIT 2001 |
03:01 min
|
|
137 |
Evaluate 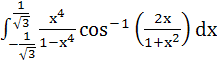
Evaluate 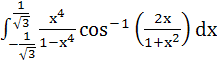
|
IIT 1995 |
09:27 min
|
|
138 |
The locus of the centre of circles which touches externally 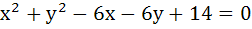 and which touches the Y-axis is given by the equation and which touches the Y-axis is given by the equation a) 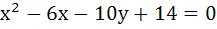 b) 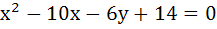 c) 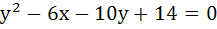 d) 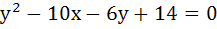
The locus of the centre of circles which touches externally 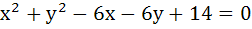 and which touches the Y-axis is given by the equation and which touches the Y-axis is given by the equation a) 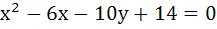 b) 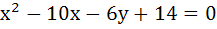 c) 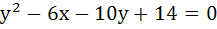 d) 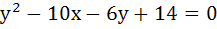
|
IIT 1993 |
04:38 min
|
|
139 |
The values of θ ε (0, 2π) for which 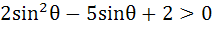 are are a) 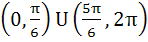 b) 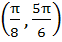 c) 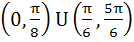 d) 
The values of θ ε (0, 2π) for which 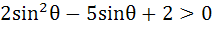 are are a) 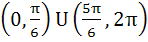 b) 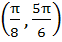 c) 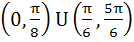 d) 
|
IIT 2006 |
03:08 min
|
|
140 |
Prove that
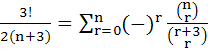
Prove that
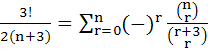
|
IIT 1997 |
09:29 min
|
|
141 |
Evaluate 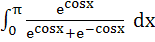 a) 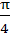 b)  c) 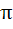 d) 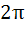
|
IIT 1999 |
01:51 min
|
|
142 |
A, B, C , D are four points in a plane with position vectors a, b, c, d respectively, such that 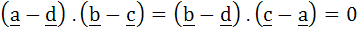 . The point D then is the . . . . . . . of the triangle ABC. . The point D then is the . . . . . . . of the triangle ABC.
A, B, C , D are four points in a plane with position vectors a, b, c, d respectively, such that 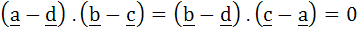 . The point D then is the . . . . . . . of the triangle ABC. . The point D then is the . . . . . . . of the triangle ABC.
|
IIT 1984 |
02:30 min
|
|
143 |
If 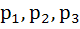 are altitudes of a triangle from the vertices A, B, C and Δ the area of the triangle then are altitudes of a triangle from the vertices A, B, C and Δ the area of the triangle then  a) True b) False
If 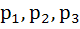 are altitudes of a triangle from the vertices A, B, C and Δ the area of the triangle then are altitudes of a triangle from the vertices A, B, C and Δ the area of the triangle then  a) True b) False
|
IIT 1978 |
03:23 min
|
|
144 |
The sum of the coefficients of the polynomial (1 + x – 3x2)2163 is
The sum of the coefficients of the polynomial (1 + x – 3x2)2163 is
|
IIT 1982 |
01:22 min
|
|
145 |
If  at x = π at x = π a)  b) π c) 2π d) 4π
If  at x = π at x = π a)  b) π c) 2π d) 4π
|
IIT 2004 |
01:14 min
|
|
146 |
If the vectors
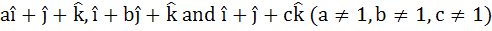 are coplanar then the value of 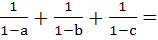 . . . . . . . . . . . .
If the vectors
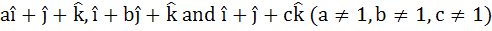 are coplanar then the value of 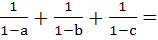 . . . . . . . . . . . .
|
IIT 1987 |
04:15 min
|
|
147 |
Let n be a positive integer. If the coefficient of the 2nd, 3rd and 4th terms in the expansion of (1 + x)n are in arithmetic progression then n = …..
Let n be a positive integer. If the coefficient of the 2nd, 3rd and 4th terms in the expansion of (1 + x)n are in arithmetic progression then n = …..
|
IIT 1994 |
03:54 min
|
|
148 |
Multiple choices If x + |y| = 2y, then y as a function of x is a) Defined for all real x b) Continuous at x = 0 c) Differentiable for all x d) Such that 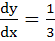 for x < 0 for x < 0
Multiple choices If x + |y| = 2y, then y as a function of x is a) Defined for all real x b) Continuous at x = 0 c) Differentiable for all x d) Such that 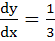 for x < 0 for x < 0
|
IIT 1984 |
03:53 min
|
|
149 |
The value of the integral 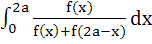 is equal to a is equal to a a) True b) False
The value of the integral 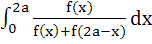 is equal to a is equal to a a) True b) False
|
IIT 1988 |
01:46 min
|
|
150 |
A unit vector coplanar with 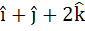 and and 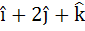 and perpendicular to and perpendicular to 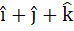 is . . . . . is . . . . .
|
IIT 1992 |
04:49 min
|