|
1076 |
One or more than one correct option A circle S passes through the point (0, 1) and is orthogonal to the circles (x – 1)2 + y2 = 16 and x2 + y2 = 1, then a) Radius of S is 8 b) Radius of S is 7 c) Centre of S is (−7, 1) d) Centre of S is (−8, 1)
One or more than one correct option A circle S passes through the point (0, 1) and is orthogonal to the circles (x – 1)2 + y2 = 16 and x2 + y2 = 1, then a) Radius of S is 8 b) Radius of S is 7 c) Centre of S is (−7, 1) d) Centre of S is (−8, 1)
|
IIT 2014 |
|
|
1077 |
The locus of the midpoint of a chord of the circle 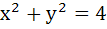 which subtend a right angle at the origin is which subtend a right angle at the origin is a) 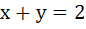 b) 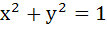 c) 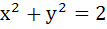 d) 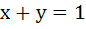
The locus of the midpoint of a chord of the circle 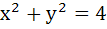 which subtend a right angle at the origin is which subtend a right angle at the origin is a) 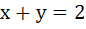 b) 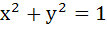 c) 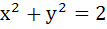 d) 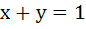
|
IIT 1984 |
|
|
1078 |
If n is a positive integer and 0 ≤ v < π then show that 
If n is a positive integer and 0 ≤ v < π then show that 
|
IIT 1994 |
|
|
1079 |
A tangent PT is drawn to the circle x2 + y2 = 4 at the point . A straight line L, perpendicular to PT is tangent to the circle (x – 3)2 + y2 = 1A possible equation of L is a) b) c) d)
A tangent PT is drawn to the circle x2 + y2 = 4 at the point . A straight line L, perpendicular to PT is tangent to the circle (x – 3)2 + y2 = 1A possible equation of L is a) b) c) d)
|
IIT 2012 |
|
|
1080 |
Let 0 < Ai < π for i = 1, 2, . . . n. Use mathematical induction to prove that
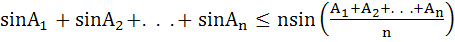
where n ≥ 1 is a natural number.
Let 0 < Ai < π for i = 1, 2, . . . n. Use mathematical induction to prove that
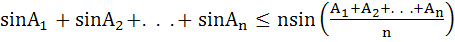
where n ≥ 1 is a natural number.
|
IIT 1997 |
|
|
1081 |
The centre of those circles which touch the circle x2 + y2 – 8x – 8y = 0, externally and also touch the X- axis, lie on a) A circle b) An ellipse which is not a circle c) A hyperbola d) A parabola
The centre of those circles which touch the circle x2 + y2 – 8x – 8y = 0, externally and also touch the X- axis, lie on a) A circle b) An ellipse which is not a circle c) A hyperbola d) A parabola
|
IIT 2016 |
|
|
1082 |
Solve 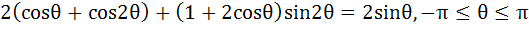
Solve 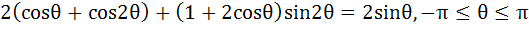
|
IIT 1978 |
|
|
1083 |
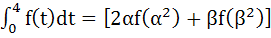 for every 0 < α, β < 2. for every 0 < α, β < 2.
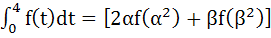 for every 0 < α, β < 2. for every 0 < α, β < 2.
|
IIT 2003 |
|
|
1084 |
Let (x, y) be any point on the parabola y2 = 4x. Let P be the point that divides the line segment from (0, 0) to (x, y) in the ratio of 1 : 3. Then the locus of P is a) x2 = y b) y2 = 2x c) y2 = x d) x2 = 2y
Let (x, y) be any point on the parabola y2 = 4x. Let P be the point that divides the line segment from (0, 0) to (x, y) in the ratio of 1 : 3. Then the locus of P is a) x2 = y b) y2 = 2x c) y2 = x d) x2 = 2y
|
IIT 2011 |
|
|
1085 |
The value of 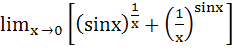 where x > 0 is where x > 0 is a) 0 b) – 1 c) 1 d) 2
The value of 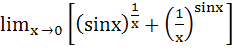 where x > 0 is where x > 0 is a) 0 b) – 1 c) 1 d) 2
|
IIT 2006 |
|
|
1086 |
The value of 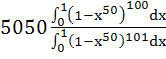 a) 5050 b) 5051 c) 100 d) 101
The value of 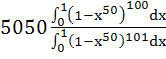 a) 5050 b) 5051 c) 100 d) 101
|
IIT 2006 |
|
|
1087 |
Let the curve C be the mirror image of the parabola y2 = 4x with respect to the line x + y + 4 = 0. If A and B are points of intersection of C with the line y = −5 then the distance between A and B is . . .?
Let the curve C be the mirror image of the parabola y2 = 4x with respect to the line x + y + 4 = 0. If A and B are points of intersection of C with the line y = −5 then the distance between A and B is . . .?
|
IIT 2015 |
|
|
1088 |
Consider the parabola y2 = 8x. Let △1 be the area of the triangle formed by the end points of its latus rectum and the point on the parabola and △2 be the area of the triangle formed by drawing tangent at P and the end points of the latus rectum. Then is
Consider the parabola y2 = 8x. Let △1 be the area of the triangle formed by the end points of its latus rectum and the point on the parabola and △2 be the area of the triangle formed by drawing tangent at P and the end points of the latus rectum. Then is
|
IIT 2011 |
|
|
1089 |
Multiple choices Let g (x) = x f (x), where 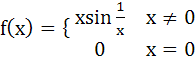 at x = 0 at x = 0 a) g is  but but  is not continuous is not continuous b) g is  while f is not while f is not c) f and g are both differentiable d) g is  and and  is continuous is continuous
Multiple choices Let g (x) = x f (x), where 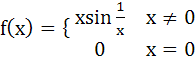 at x = 0 at x = 0 a) g is  but but 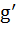 is not continuous is not continuous b) g is  while f is not while f is not c) f and g are both differentiable d) g is  and and 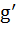 is continuous is continuous
|
IIT 1994 |
|
|
1090 |
A five digit number divisible by 3 is formed using the numerals 0, 1, 2, 3, 4, and 5 without repetition. Total number of ways this can be done is a) At least 30 b) At most 20 c) Exactly 25 d) None of these
A five digit number divisible by 3 is formed using the numerals 0, 1, 2, 3, 4, and 5 without repetition. Total number of ways this can be done is a) At least 30 b) At most 20 c) Exactly 25 d) None of these
|
IIT 1989 |
|
|
1091 |
A rectangle with sides (2m – 1) and (2n – 1) is divided into squares of unit length by drawing parallel lines. Then the number of rectangles possible with odd side lengths is a) mn (m + 1)(n + 1) b) 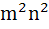 c)  d) 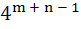
A rectangle with sides (2m – 1) and (2n – 1) is divided into squares of unit length by drawing parallel lines. Then the number of rectangles possible with odd side lengths is a) mn (m + 1)(n + 1) b) 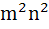 c)  d) 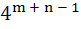
|
IIT 2005 |
|
|
1092 |
If the normal to the curve y = f(x) at the point (3, 4) makes an angle 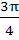 with the positive X–axis then with the positive X–axis then 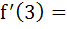 a) – 1 b) 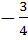 c)  d) 1
If the normal to the curve y = f(x) at the point (3, 4) makes an angle 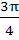 with the positive X–axis then with the positive X–axis then 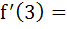 a) – 1 b) 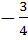 c)  d) 1
|
IIT 2000 |
|
|
1093 |
A circle passes through points A, B and C with the line segment AC as its diameter. A line passing through A intersects the chord BC at D inside the circle. If ∠DAB and ∠CAB are α and β respectively and the distance between the point A and the midpoint of the line segment DC is d, prove that the area of the circle is
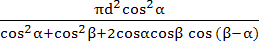
A circle passes through points A, B and C with the line segment AC as its diameter. A line passing through A intersects the chord BC at D inside the circle. If ∠DAB and ∠CAB are α and β respectively and the distance between the point A and the midpoint of the line segment DC is d, prove that the area of the circle is
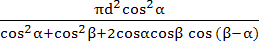
|
IIT 1996 |
|
|
1094 |
Domain of definition of the function f (x) =  for real valued x is for real valued x is a) 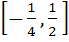 b) 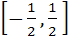 c)  d) 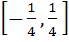
Domain of definition of the function f (x) =  for real valued x is for real valued x is a) 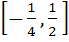 b) 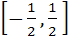 c)  d) 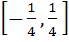
|
IIT 2003 |
|
|
1095 |
Find the values of a and b, so that the functions 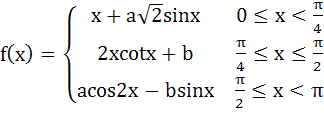
Is continuous for 0 ≤ x ≤ π a) 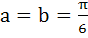 b) 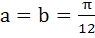 c) 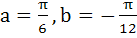 d) 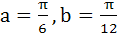
Find the values of a and b, so that the functions 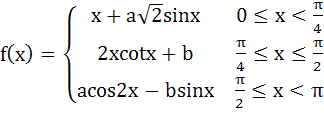
Is continuous for 0 ≤ x ≤ π a) 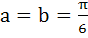 b) 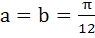 c) 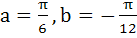 d) 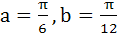
|
IIT 1989 |
|
|
1096 |
C1 and C2 are two concentric circles, the radius of C2 being twice of C1 . From a point on C2 tangents PA and PB are drawn to C1. Prove that the centroid of ΔPAB lies on C1.
C1 and C2 are two concentric circles, the radius of C2 being twice of C1 . From a point on C2 tangents PA and PB are drawn to C1. Prove that the centroid of ΔPAB lies on C1.
|
IIT 1998 |
|
|
1097 |
In [0, 1], Lagrange’s Mean Value theorem is not applicable to a) 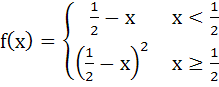 b) 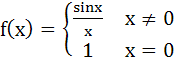 c)  d) 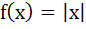
In [0, 1], Lagrange’s Mean Value theorem is not applicable to a) 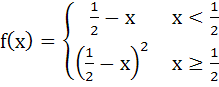 b) 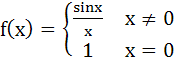 c)  d) 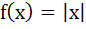
|
IIT 2003 |
|
|
1098 |
Let α ε ℝ, then a function f : ℝ → ℝ is differentiable at α if and only if there is a function g : ℝ → ℝ which is continuous at α and satisfies f(x) – f(α) = g(x) (x – α) for all x ε ℝ. a) True b) False
Let α ε ℝ, then a function f : ℝ → ℝ is differentiable at α if and only if there is a function g : ℝ → ℝ which is continuous at α and satisfies f(x) – f(α) = g(x) (x – α) for all x ε ℝ. a) True b) False
|
IIT 2001 |
|
|
1099 |
The area bounded by the angle bisectors of the lines x2 – y2 + 2y = 1 and the line x + y = 3 is a) 2 b) 3 c) 4 d) 6
The area bounded by the angle bisectors of the lines x2 – y2 + 2y = 1 and the line x + y = 3 is a) 2 b) 3 c) 4 d) 6
|
IIT 2004 |
|
|
1100 |
If two functions f and g satisfy the given conditions 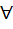 x, y ε ℝ, f(x – y) = f(x)g(y) – f(y)g(x) and g(x – y) = g(x) . g(y) + f(x) . f(y). x, y ε ℝ, f(x – y) = f(x)g(y) – f(y)g(x) and g(x – y) = g(x) . g(y) + f(x) . f(y). If the RHD at x = 0 exists for f(x) then find the derivative of g(x) at x = 0.
If two functions f and g satisfy the given conditions 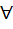 x, y ε ℝ, f(x – y) = f(x)g(y) – f(y)g(x) and g(x – y) = g(x) . g(y) + f(x) . f(y). x, y ε ℝ, f(x – y) = f(x)g(y) – f(y)g(x) and g(x – y) = g(x) . g(y) + f(x) . f(y). If the RHD at x = 0 exists for f(x) then find the derivative of g(x) at x = 0.
|
IIT 2005 |
|