|
1001 |
Prove that 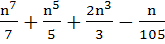 is an integer for every positive integer. is an integer for every positive integer.
Prove that 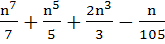 is an integer for every positive integer. is an integer for every positive integer.
|
IIT 1990 |
|
|
1002 |
If f is a continuous function with 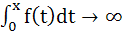 as |x| → ∞ then show that every line y = mx intersects the curve as |x| → ∞ then show that every line y = mx intersects the curve  . .
If f is a continuous function with 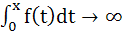 as |x| → ∞ then show that every line y = mx intersects the curve as |x| → ∞ then show that every line y = mx intersects the curve  . .
|
IIT 1991 |
|
|
1003 |
Show, by vector method, that the angular bisectors of a triangle are concurrent and find an expression for the position vector of point of concurrency in terms of position vectors of the vertices.
Show, by vector method, that the angular bisectors of a triangle are concurrent and find an expression for the position vector of point of concurrency in terms of position vectors of the vertices.
|
IIT 2001 |
|
|
1004 |
The C be a circle with the centre at (1, 1) and radius 1. If T is the circle centred at (0, k) passing through origin and touches the circle C externally, then the radius of T is equal to a) b) c) d)
The C be a circle with the centre at (1, 1) and radius 1. If T is the circle centred at (0, k) passing through origin and touches the circle C externally, then the radius of T is equal to a) b) c) d)
|
IIT 2014 |
|
|
1005 |
If AB is a diameter of a circle and C is any point on the circumference of the circle then a) The area of ΔABC is maximum when it is isosceles b) The area of ΔABC is minimum when it is isosceles c) The perimeter of ΔABC is minimum when it is isosceles d) None of these
If AB is a diameter of a circle and C is any point on the circumference of the circle then a) The area of ΔABC is maximum when it is isosceles b) The area of ΔABC is minimum when it is isosceles c) The perimeter of ΔABC is minimum when it is isosceles d) None of these
|
IIT 1983 |
|
|
1006 |
Let f : ℝ → ℝ be any function. Define g : ℝ → ℝ by g (x) = |f (x)| for all x. Then g is a) Onto if f is onto b) One to one if f is one to one c) Continuous if f is continuous d) Differentiable if f is differentiable
Let f : ℝ → ℝ be any function. Define g : ℝ → ℝ by g (x) = |f (x)| for all x. Then g is a) Onto if f is onto b) One to one if f is one to one c) Continuous if f is continuous d) Differentiable if f is differentiable
|
IIT 2000 |
|
|
1007 |
Evaluate 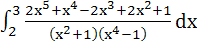 a) 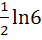 b) 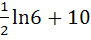 c) 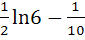 d) 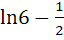
|
IIT 1993 |
|
|
1008 |
One or more than one correct option The circle C1 : x2 + y2 = 3 with centre at O intersect the parabola x2 = 2y at the point P in the first quadrant. Let the tangent to the circle C1 at P touches other two circles C2 and C3 at R2 and R3 respectively. Suppose C2 and C3 have equal radii and centres Q2 and Q3 respectively. If Q2 and Q3 lie on the Y- axis, then a) b) c) d)
One or more than one correct option The circle C1 : x2 + y2 = 3 with centre at O intersect the parabola x2 = 2y at the point P in the first quadrant. Let the tangent to the circle C1 at P touches other two circles C2 and C3 at R2 and R3 respectively. Suppose C2 and C3 have equal radii and centres Q2 and Q3 respectively. If Q2 and Q3 lie on the Y- axis, then a) b) c) d)
|
IIT 2016 |
|
|
1009 |
Let f : ℝ → ℝ be a function defined by f (x) = 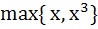 . The set of points where f (x) is not differentiable is . The set of points where f (x) is not differentiable is a)  } } b) 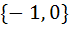 c) {0, 1} d) 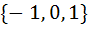
Let f : ℝ → ℝ be a function defined by f (x) = 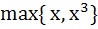 . The set of points where f (x) is not differentiable is . The set of points where f (x) is not differentiable is a)  } } b) 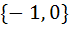 c) {0, 1} d) 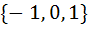
|
IIT 2001 |
|
|
1010 |
The circle passing through the point (−1, 0) and touching the Y – axis at (0, 2) also passes through the point a) b) c) d)
The circle passing through the point (−1, 0) and touching the Y – axis at (0, 2) also passes through the point a) b) c) d)
|
IIT 2011 |
|
|
1011 |
Let a, b, c be positive real numbers such that b2 – 4ac > 0 and let α1 = c. Prove by induction that
 Is well defined and  for n=1, 2, … for n=1, 2, … Here well defined means that the denominator in the expression of 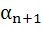 is not zero. is not zero.
Let a, b, c be positive real numbers such that b2 – 4ac > 0 and let α1 = c. Prove by induction that
 Is well defined and  for n=1, 2, … for n=1, 2, … Here well defined means that the denominator in the expression of 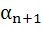 is not zero. is not zero.
|
IIT 2001 |
|
|
1012 |
Let O be the vertex and Q be any point on the parabola x2 = 8y. If the point P divides the line segment internally in the ratio 1 : 3 then the locus of P is a) x2 = y b) y2 = x c) y2 = 2x d) x2 = 2y
Let O be the vertex and Q be any point on the parabola x2 = 8y. If the point P divides the line segment internally in the ratio 1 : 3 then the locus of P is a) x2 = y b) y2 = x c) y2 = 2x d) x2 = 2y
|
IIT 2015 |
|
|
1013 |
Solve the following equation for x
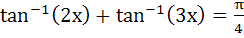 a) −1 b)  c) 0 d) −1 and 
Solve the following equation for x
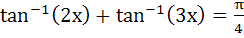 a) −1 b)  c) 0 d) −1 and 
|
IIT 1978 |
|
|
1014 |
If f is a differentiable function satisfying  for all n ≥ 1, for all n ≥ 1, n 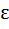 I then I then a) 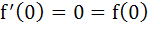 b) 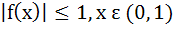 c) 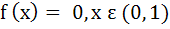 d) 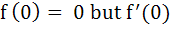 is not necessarily zero is not necessarily zero
If f is a differentiable function satisfying  for all n ≥ 1, for all n ≥ 1, n 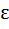 I then I then a) 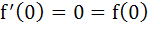 b) 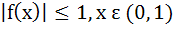 c) 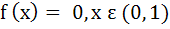 d) 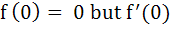 is not necessarily zero is not necessarily zero
|
IIT 2005 |
|
|
1015 |
Evaluate 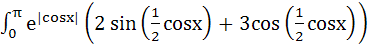
Evaluate 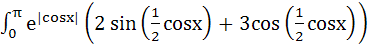
|
IIT 2005 |
|
|
1016 |
Let S be the focus of the parabola y2 = 8x and PQ be the common chord of the circle x2 + y2 – 2x – 4y = 0 and the given parabola. The area of △QPS is a) 2 sq. units b) 4 sq. units c) 6 sq. units d) 8 sq. units
Let S be the focus of the parabola y2 = 8x and PQ be the common chord of the circle x2 + y2 – 2x – 4y = 0 and the given parabola. The area of △QPS is a) 2 sq. units b) 4 sq. units c) 6 sq. units d) 8 sq. units
|
IIT 2012 |
|
|
1017 |
Multiple choices The function f (x) = 1 + |sinx| is a) continuous nowhere b) continuous everywhere c) differentiable nowhere d) not differentiable at x = 0 e) not differentiable at infinite number of points
Multiple choices The function f (x) = 1 + |sinx| is a) continuous nowhere b) continuous everywhere c) differentiable nowhere d) not differentiable at x = 0 e) not differentiable at infinite number of points
|
IIT 1986 |
|
|
1018 |
Let a, r, s, t be non-zero real numbers. Let P(at2, 2at), Q, R(ar2, 2ar and S(as2, 2as) be distinct points on the parabola y2 = 4ax. Suppose PQ is the focal chord and QR and PK are parallel, where K is point (2a, 0)If st = 1 then the tangent at P and normal at S to the parabola meet at a point whose ordinate is a) b) c) d)
Let a, r, s, t be non-zero real numbers. Let P(at2, 2at), Q, R(ar2, 2ar and S(as2, 2as) be distinct points on the parabola y2 = 4ax. Suppose PQ is the focal chord and QR and PK are parallel, where K is point (2a, 0)If st = 1 then the tangent at P and normal at S to the parabola meet at a point whose ordinate is a) b) c) d)
|
IIT 2014 |
|
|
1019 |
The tangent PT and the normal PN of the parabola y2 = 4ax at the point P on it meet its axis at the points T and N respectively. The locus of the centroid of the triangle PTM is a parabola whose a) Vertex is b) Directrix is x = 0 c) Latus rectum is d) Focus is (a, 0)
The tangent PT and the normal PN of the parabola y2 = 4ax at the point P on it meet its axis at the points T and N respectively. The locus of the centroid of the triangle PTM is a parabola whose a) Vertex is b) Directrix is x = 0 c) Latus rectum is d) Focus is (a, 0)
|
IIT 2009 |
|
|
1020 |
Let f and g be increasing and decreasing functions, respectively from [0, ∞) to [0, ∞). Let h(x) =f(g(x)). If h(0) = 0 then h(x) – h(t) is a) Always zero b) Always negative c) Always positive d) Strictly increasing e) None of these
Let f and g be increasing and decreasing functions, respectively from [0, ∞) to [0, ∞). Let h(x) =f(g(x)). If h(0) = 0 then h(x) – h(t) is a) Always zero b) Always negative c) Always positive d) Strictly increasing e) None of these
|
IIT 1988 |
|
|
1021 |
Let E = {1, 2, 3, 4} and F = {1, 2} then the number of onto functions from E to F is a) 14 b) 16 c) 12 d) 8
Let E = {1, 2, 3, 4} and F = {1, 2} then the number of onto functions from E to F is a) 14 b) 16 c) 12 d) 8
|
IIT 2001 |
|
|
1022 |
On the interval [0, 1] the function 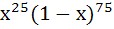 takes the maximum value at the point takes the maximum value at the point a) 0 b)  c)  d) 
On the interval [0, 1] the function 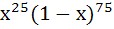 takes the maximum value at the point takes the maximum value at the point a) 0 b)  c)  d) 
|
IIT 1995 |
|
|
1023 |
Let f (x) be continuous and g (x) be a discontinuous function. Prove that f (x) + g (x) is a discontinuous function. a) True b) False c) Could be continuous or discontinuous
Let f (x) be continuous and g (x) be a discontinuous function. Prove that f (x) + g (x) is a discontinuous function. a) True b) False c) Could be continuous or discontinuous
|
IIT 1987 |
|
|
1024 |
Find the coordinates of the point at which the circles x2 + y2 – 4x – 2y = – 4 and x2 + y2 – 12x – 8y = – 36 touch each other. Also find the equation of the common tangents touching the circles at distinct points.
Find the coordinates of the point at which the circles x2 + y2 – 4x – 2y = – 4 and x2 + y2 – 12x – 8y = – 36 touch each other. Also find the equation of the common tangents touching the circles at distinct points.
|
IIT 1993 |
|
|
1025 |
Draw the graph of the function y = [x] + |1 – x|, – 1 ≤ x ≤ 3. Determine the points, if any, where the function is not differentiable. a) y is differentiable everywhere b) y is not differentiable at x = 0 c) y is not differentiable at x = 0, 1, 2 d) y is not differentiable at x = 0, 1, 2 and 3
Draw the graph of the function y = [x] + |1 – x|, – 1 ≤ x ≤ 3. Determine the points, if any, where the function is not differentiable. a) y is differentiable everywhere b) y is not differentiable at x = 0 c) y is not differentiable at x = 0, 1, 2 d) y is not differentiable at x = 0, 1, 2 and 3
|
IIT 1989 |
|