|
776 |
If A > 0, B > 0 and A + B =  , then the maximum value of tan A tanB is ………. , then the maximum value of tan A tanB is ………. a)  b)  c)  d) 
If A > 0, B > 0 and A + B =  , then the maximum value of tan A tanB is ………. , then the maximum value of tan A tanB is ………. a)  b)  c)  d) 
|
IIT 1993 |
|
|
777 |
Let 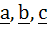 be non–coplanar unit vectors equally inclined to one another at an angle θ. If be non–coplanar unit vectors equally inclined to one another at an angle θ. If 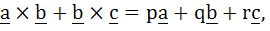 find p, q, r in terms of θ find p, q, r in terms of θ
Let 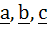 be non–coplanar unit vectors equally inclined to one another at an angle θ. If be non–coplanar unit vectors equally inclined to one another at an angle θ. If 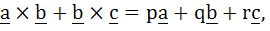 find p, q, r in terms of θ find p, q, r in terms of θ
|
IIT 1997 |
|
|
778 |
If 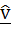 is the unit vector along the incident ray, is the unit vector along the incident ray, 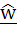 is a unit vector along the reflected ray and is a unit vector along the reflected ray and 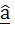 is a unit vector along the outward drawn normal to the plane mirror at the point of incidence. Find is a unit vector along the outward drawn normal to the plane mirror at the point of incidence. Find 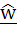 in terms of in terms of 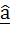 and and 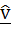
|
IIT 2005 |
|
|
779 |
True / False For any three vectors a, b and c
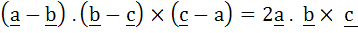 a) True b) False
True / False For any three vectors a, b and c
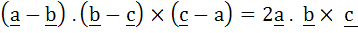 a) True b) False
|
IIT 1989 |
|
|
780 |
Multiple choices
For a positive integer n, let
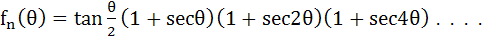
. . .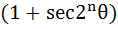 then then a) 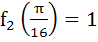 b) 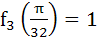 c) 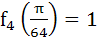 d) 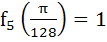
Multiple choices
For a positive integer n, let
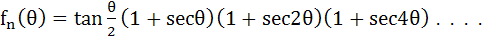
. . .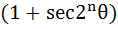 then then a) 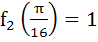 b) 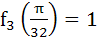 c) 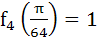 d) 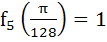
|
IIT 1999 |
|
|
781 |
For all 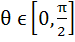 , , 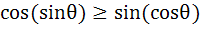 a) True b) False
For all 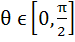 , , 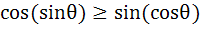 a) True b) False
|
IIT 1981 |
|
|
782 |
Let f (x) = |x – 1| then a) f (x2) = |f (x)|2 b) f (x + y) = f (x) + f (y) c) f (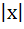 ) = |f (x)| ) = |f (x)| d) None of these
Let f (x) = |x – 1| then a) f (x2) = |f (x)|2 b) f (x + y) = f (x) + f (y) c) f (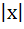 ) = |f (x)| ) = |f (x)| d) None of these
|
IIT 1983 |
|
|
783 |
Let the vectors  represent the edges of a regular hexagon represent the edges of a regular hexagon Statement 1 - 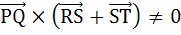 because because Statement 2 - 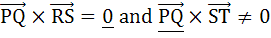 a) Statement 1 and 2 are true and Statement 2 is a correct explanation of statement 1. b) Statement 1 and 2 are true and Statement 2 is not a correct explanation of statement 1. c) Statement 1 is true. Statement 2 is false. d) Statement 1 is false. Statement 2 is true.
Let the vectors  represent the edges of a regular hexagon represent the edges of a regular hexagon Statement 1 - 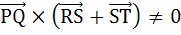 because because Statement 2 - 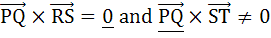 a) Statement 1 and 2 are true and Statement 2 is a correct explanation of statement 1. b) Statement 1 and 2 are true and Statement 2 is not a correct explanation of statement 1. c) Statement 1 is true. Statement 2 is false. d) Statement 1 is false. Statement 2 is true.
|
IIT 2007 |
|
|
784 |
Find the smallest possible value of p for which the equation
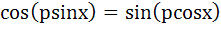 a) 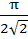 b) 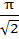 c)  d) 
Find the smallest possible value of p for which the equation
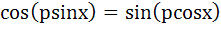 a) 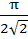 b) 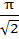 c)  d) 
|
IIT 1995 |
|
|
785 |
If f (x) = 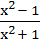 for every real x then the minimum value of f for every real x then the minimum value of f a) does not exist because f is unbounded b) is not attained even though f is bounded c) is equal to 1 d) is equal to −1
If f (x) = 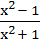 for every real x then the minimum value of f for every real x then the minimum value of f a) does not exist because f is unbounded b) is not attained even though f is bounded c) is equal to 1 d) is equal to −1
|
IIT 1998 |
|
|
786 |
Find the larger of cos(lnθ) and ln(cosθ) if  < θ < < θ <  . . a) cos(lnθ) b) ln(cosθ) c) Neither is larger throughout the interval
Find the larger of cos(lnθ) and ln(cosθ) if  < θ < < θ <  . . a) cos(lnθ) b) ln(cosθ) c) Neither is larger throughout the interval
|
IIT 1983 |
|
|
787 |
If the function f : [ 1, 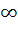 ) → [ 1, ) → [ 1, 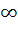 ) is defined by f (x) = 2x(x – 1) then ) is defined by f (x) = 2x(x – 1) then
f -1(x) is a) 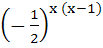 b)  ( (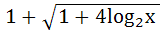 ) ) c)  ( (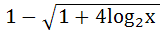 ) ) d) 
|
IIT 1999 |
|
|
788 |
If 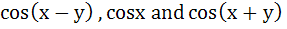 are in harmonic progression then are in harmonic progression then  ………… ………… a) 1 b) 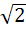 c) 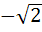 d) 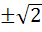
If 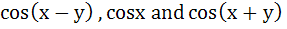 are in harmonic progression then are in harmonic progression then  ………… ………… a) 1 b) 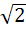 c) 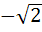 d) 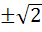
|
IIT 1997 |
|
|
789 |
If 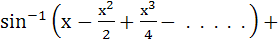 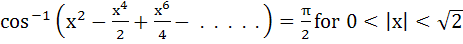
then x equals a)  b) 1 c) 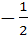 d) –1
If 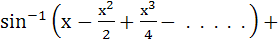 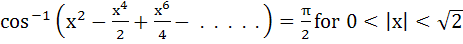
then x equals a)  b) 1 c) 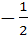 d) –1
|
IIT 1999 |
|
|
790 |
Let f ( x ) =  , x ≠ , x ≠ 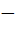 1 then for what value of a is f ( f (x)) = x 1 then for what value of a is f ( f (x)) = x a) 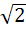 b) 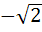 c) 1 d) 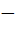 1 1
Let f ( x ) =  , x ≠ , x ≠ 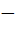 1 then for what value of a is f ( f (x)) = x 1 then for what value of a is f ( f (x)) = x a) 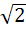 b) 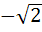 c) 1 d) 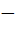 1 1
|
IIT 2001 |
|
|
791 |
If f : [ 0,  ) )  [ 0, [ 0,  ) and f (x) = ) and f (x) = 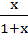 then f is then f is a) one-one and onto b) one-one but not onto c) onto but not one-one d) neither one-one nor onto
If f : [ 0,  ) )  [ 0, [ 0,  ) and f (x) = ) and f (x) = 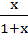 then f is then f is a) one-one and onto b) one-one but not onto c) onto but not one-one d) neither one-one nor onto
|
IIT 2003 |
|
|
792 |
Match the following Let (x, y) be such that  = = 
| Column 1 | Column 2 | | i) If a=1 and b=0 then (x, y) | A)Lies on the circle
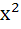 + +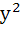 =1 =1 | | ii) If a=1 and b=1 then (x, y) | B)Lies on
(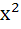 −1)( −1)(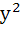 −1) = 0 −1) = 0 | | iii) If a=1 and b=2 then (x, y) | C)Lies on y = x | | iv) If a=2 and b=2 then (x, y) | D)Lies on
(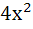 −1)( −1)(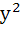 −1) = 0 −1) = 0 |
Match the following Let (x, y) be such that  = = 
| Column 1 | Column 2 | | i) If a=1 and b=0 then (x, y) | A)Lies on the circle
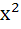 + +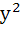 =1 =1 | | ii) If a=1 and b=1 then (x, y) | B)Lies on
(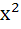 −1)( −1)(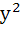 −1) = 0 −1) = 0 | | iii) If a=1 and b=2 then (x, y) | C)Lies on y = x | | iv) If a=2 and b=2 then (x, y) | D)Lies on
(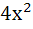 −1)( −1)(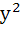 −1) = 0 −1) = 0 |
|
IIT 2007 |
|
|
793 |
f (x) = 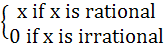
and g (x) = 
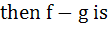 a) neither one-one nor onto b) one-one and onto c) one-one and into d) many one and onto
f (x) = 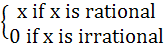
and g (x) = 
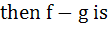 a) neither one-one nor onto b) one-one and onto c) one-one and into d) many one and onto
|
IIT 2005 |
|
|
794 |
Let O (0, 0), P (3, 4), Q (6, 0) be the vertices of the triangle OPQ. The point inside the triangle OPQ is such that OPR, PQR, OQR are of equal area. The coordinates of R are a) 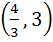 b) 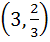 c) 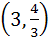 d) 
Let O (0, 0), P (3, 4), Q (6, 0) be the vertices of the triangle OPQ. The point inside the triangle OPQ is such that OPR, PQR, OQR are of equal area. The coordinates of R are a) 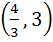 b) 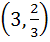 c) 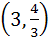 d) 
|
IIT 2006 |
|
|
795 |
If f be a one–one function with domain { x, y, z}and range { 1, 2, 3}. It is given that exactly one of the following statements is true and the remaining statements are false. Determine 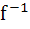 (1) (1) 1. f(x) = 1 2. f(y) ≠ 1 3. f(z) ≠ 2 a) {0} b) {1} c) {y} d) none of the above
If f be a one–one function with domain { x, y, z}and range { 1, 2, 3}. It is given that exactly one of the following statements is true and the remaining statements are false. Determine 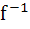 (1) (1) 1. f(x) = 1 2. f(y) ≠ 1 3. f(z) ≠ 2 a) {0} b) {1} c) {y} d) none of the above
|
IIT 1982 |
|
|
796 |
One or more correct answers
In triangle ABC the internal angle bisector of ∠A meets the side BC in D. DE is a perpendicular to AD which meets AC in E and AB in F. Then a) AE is harmonic mean of b and c b) AD 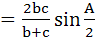 c) 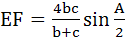 d) Δ AEF is isosceles
One or more correct answers
In triangle ABC the internal angle bisector of ∠A meets the side BC in D. DE is a perpendicular to AD which meets AC in E and AB in F. Then a) AE is harmonic mean of b and c b) AD 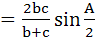 c) 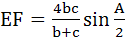 d) Δ AEF is isosceles
|
IIT 2006 |
|
|
797 |
For a triangle ABC it is given that 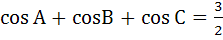 , then Δ ABC is equilateral. , then Δ ABC is equilateral. a) True b) False
For a triangle ABC it is given that 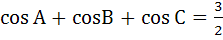 , then Δ ABC is equilateral. , then Δ ABC is equilateral. a) True b) False
|
IIT 1984 |
|
|
798 |
True / False The function f (x) = 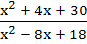 is not one to one. is not one to one. a) True b) False
True / False The function f (x) = 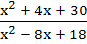 is not one to one. is not one to one. a) True b) False
|
IIT 1983 |
|
|
799 |
Find the set of all values of a such that 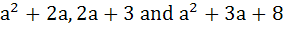 are sides of a triangle. are sides of a triangle. a) (0, 3) b) (3, ∞) c) (0, 5) d) (5, ∞)
Find the set of all values of a such that 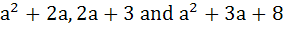 are sides of a triangle. are sides of a triangle. a) (0, 3) b) (3, ∞) c) (0, 5) d) (5, ∞)
|
IIT 1985 |
|
|
800 |
Fill in the blank Let A be the set of n distinct elements then the total number of distinct functions from A to A is ……… and out of these …… are onto a) n!, 1 b) nn, n! c) nn, 1 d) none of the above
Fill in the blank Let A be the set of n distinct elements then the total number of distinct functions from A to A is ……… and out of these …… are onto a) n!, 1 b) nn, n! c) nn, 1 d) none of the above
|
IIT 1985 |
|