|
601 |
If  has its extremum value at x = has its extremum value at x =  1 and x = 2, then 1 and x = 2, then a) a = 2, b =  1 1 b) a = 2,  c) a =  2, 2, 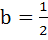 d) None of these
If  has its extremum value at x = has its extremum value at x =  1 and x = 2, then 1 and x = 2, then a) a = 2, b =  1 1 b) a = 2,  c) a =  2, 2, 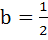 d) None of these
|
IIT 1983 |
02:13 min
|
|
602 |
Lines 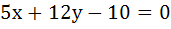 and and  touch a circle C1 of diameter 6. If the centre of C1 lies in the first quadrant, find the equation of the circle C2 which is concentric with C1 and cuts intercepts of length 8 on these lines. touch a circle C1 of diameter 6. If the centre of C1 lies in the first quadrant, find the equation of the circle C2 which is concentric with C1 and cuts intercepts of length 8 on these lines.
Lines 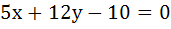 and and  touch a circle C1 of diameter 6. If the centre of C1 lies in the first quadrant, find the equation of the circle C2 which is concentric with C1 and cuts intercepts of length 8 on these lines. touch a circle C1 of diameter 6. If the centre of C1 lies in the first quadrant, find the equation of the circle C2 which is concentric with C1 and cuts intercepts of length 8 on these lines.
|
IIT 1986 |
07:04 min
|
|
603 |
The solution set of the equations  where x and y are real is …………. where x and y are real is …………. a) 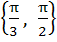 b) 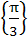 c) 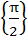 d) No solution
The solution set of the equations  where x and y are real is …………. where x and y are real is …………. a) 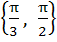 b) 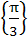 c) 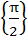 d) No solution
|
IIT 1986 |
02:21 min
|
|
604 |
If f (θ) = sinθ (sinθ + sin3θ) then f (θ) a) ≥ 0 only when θ ≥ 0 b) ≤ 0 for all real θ c) ≥ 0 for all real θ d) ≤ θ only when θ ≤ 0
If f (θ) = sinθ (sinθ + sin3θ) then f (θ) a) ≥ 0 only when θ ≥ 0 b) ≤ 0 for all real θ c) ≥ 0 for all real θ d) ≤ θ only when θ ≤ 0
|
IIT 2000 |
01:05 min
|
|
605 |
Evaluate 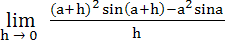 a) 2asina b) a2cosa c) 2asina + a2cosa d) 2a
Evaluate 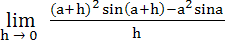 a) 2asina b) a2cosa c) 2asina + a2cosa d) 2a
|
IIT 1980 |
01:38 min
|
|
606 |
For 2 ≤ r ≤ n, 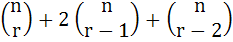 is equal to is equal to a) 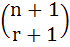 b) 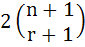 c) 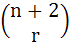 d) 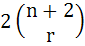
For 2 ≤ r ≤ n, 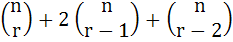 is equal to is equal to a) 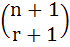 b) 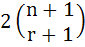 c) 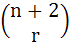 d) 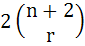
|
IIT 2000 |
03:00 min
|
|
607 |
Which of the following curves cut the parabola 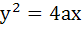 at right angles? at right angles? a)  b) 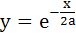 c)  d) 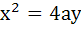
Which of the following curves cut the parabola 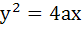 at right angles? at right angles? a)  b) 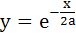 c)  d) 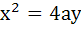
|
IIT 1994 |
02:31 min
|
|
608 |
If 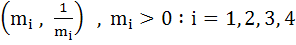 are four points on a circle then show that are four points on a circle then show that  . .
If 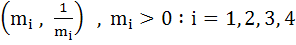 are four points on a circle then show that are four points on a circle then show that  . .
|
IIT 1989 |
01:43 min
|
|
609 |
The value of 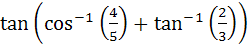 is is a) 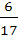 b)  c)  d) None of these
The value of 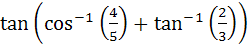 is is a) 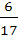 b)  c)  d) None of these
|
IIT 1983 |
02:14 min
|
|
610 |
The domain of f (x) = 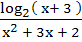 is is a) R – { 1, 1, 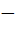 2} 2} b) (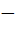 2, 2, 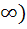 c) R – { 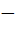 1, 1, 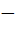 2, 2, 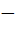 3} 3} d) (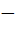 3, 3, 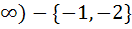
|
IIT 2001 |
01:19 min
|
|
611 |
Let 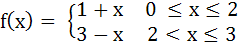 Determine the function g (x) = f (f(x)) and hence find the points of discontinuity of g if any. a) g(x) is continuous for all x except x = 1 and x = 2 b) g(x) is continuous for all x except x = 1 c) g(x) is continuous for all x except x = 2 d) g(x) is continuous for all x
Let 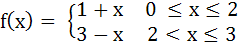 Determine the function g (x) = f (f(x)) and hence find the points of discontinuity of g if any. a) g(x) is continuous for all x except x = 1 and x = 2 b) g(x) is continuous for all x except x = 1 c) g(x) is continuous for all x except x = 2 d) g(x) is continuous for all x
|
IIT 1983 |
05:15 min
|
|
612 |
The slope of the tangent to the curve y = f(x) at [x, f(x)] is 2x + 1. The curve passes through (1, 2), then the area bounded by the curve and X–axis, and the line x = 1 is a)  b)  c)  d) 6
The slope of the tangent to the curve y = f(x) at [x, f(x)] is 2x + 1. The curve passes through (1, 2), then the area bounded by the curve and X–axis, and the line x = 1 is a)  b)  c)  d) 6
|
IIT 1995 |
03:15 min
|
|
613 |
Three circles touch each other externally. The tangents at their points of contact meet at a point whose distance from a point of contact is 4. Find the ratio of the product of the radii to the sum of the radii of the circles.
Three circles touch each other externally. The tangents at their points of contact meet at a point whose distance from a point of contact is 4. Find the ratio of the product of the radii to the sum of the radii of the circles.
|
IIT 1992 |
07:55 min
|
|
614 |
The number of solutions of 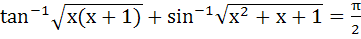 is is a) 0 b) One c) Two d) Infinite
The number of solutions of 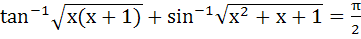 is is a) 0 b) One c) Two d) Infinite
|
IIT 2001 |
04:00 min
|
|
615 |
Let f (x) be a continuous function satisfying 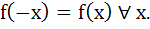 If If 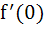 exists, find its value. exists, find its value. a) 0 b) 1 c) 2 d) 4
Let f (x) be a continuous function satisfying 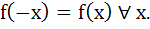 If If 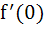 exists, find its value. exists, find its value. a) 0 b) 1 c) 2 d) 4
|
IIT 1987 |
03:18 min
|
|
616 |
The letters of the word COCHIN are permuted and all permutations are arranged in an alphabetical order as in the English dictionary. The number of words that appear before the word COCHIN is a) 360 b) 192 c) 96 d) 48
The letters of the word COCHIN are permuted and all permutations are arranged in an alphabetical order as in the English dictionary. The number of words that appear before the word COCHIN is a) 360 b) 192 c) 96 d) 48
|
IIT 2007 |
03:06 min
|
|
617 |
Five balls of different colours are to be placed in three boxes of different sizes. Each box can hold all five balls. In how many different ways can we place the balls so that no box is empty?
Five balls of different colours are to be placed in three boxes of different sizes. Each box can hold all five balls. In how many different ways can we place the balls so that no box is empty?
|
IIT 1981 |
07:04 min
|
|
618 |
If 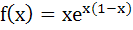 then f(x) is then f(x) is a) Increasing on 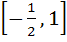 b) Decreasing on ℝ c) Increasing on ℝ d) Decreasing on 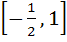
If 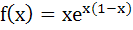 then f(x) is then f(x) is a) Increasing on 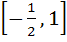 b) Decreasing on ℝ c) Increasing on ℝ d) Decreasing on 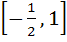
|
IIT 2001 |
02:04 min
|
|
619 |
In a triangle ABC, ∠ B =  , ∠ C = , ∠ C = 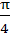 . Let D divides BC internally in the ratio 1:3 then . Let D divides BC internally in the ratio 1:3 then 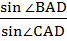 is equal to is equal to a)  b)  c) 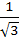 d) 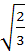
In a triangle ABC, ∠ B =  , ∠ C = , ∠ C = 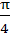 . Let D divides BC internally in the ratio 1:3 then . Let D divides BC internally in the ratio 1:3 then 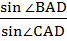 is equal to is equal to a)  b)  c) 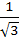 d) 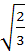
|
IIT 1995 |
03:14 min
|
|
620 |
Let  Test whether f(x) is continuous at x = 0 f(x) is differentiable at x = 0 a) f(x) is differentiable and continuous at x = 0 b) f(x) is continuous but not differentiable at x = 0 c) f(x) is neither continuous nor differentiable at x = 0
Let  Test whether f(x) is continuous at x = 0 f(x) is differentiable at x = 0 a) f(x) is differentiable and continuous at x = 0 b) f(x) is continuous but not differentiable at x = 0 c) f(x) is neither continuous nor differentiable at x = 0
|
IIT 1994 |
05:27 min
|
|
621 |
A student is allowed to select at most n books from a collection of (2n + 1) books. If the total number of ways in which he can select at least one book is 63, find the value of n?
A student is allowed to select at most n books from a collection of (2n + 1) books. If the total number of ways in which he can select at least one book is 63, find the value of n?
|
IIT 1987 |
06:50 min
|
|
622 |
Let 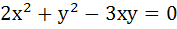 be the equation of pair of tangents from the origin O to a circle of radius 3 with centre in the first quadrant. If A is a point of contact, find the length of OA. be the equation of pair of tangents from the origin O to a circle of radius 3 with centre in the first quadrant. If A is a point of contact, find the length of OA.
Let 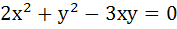 be the equation of pair of tangents from the origin O to a circle of radius 3 with centre in the first quadrant. If A is a point of contact, find the length of OA. be the equation of pair of tangents from the origin O to a circle of radius 3 with centre in the first quadrant. If A is a point of contact, find the length of OA.
|
IIT 2001 |
04:52 min
|
|
623 |
If the angles of a triangle are in the ratio 4:1:1 then the ratio of the longest side to the perimeter is a) 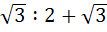 b) 1 : 6 c) 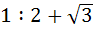 d) 2 : 3
If the angles of a triangle are in the ratio 4:1:1 then the ratio of the longest side to the perimeter is a) 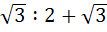 b) 1 : 6 c) 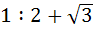 d) 2 : 3
|
IIT 2003 |
03:18 min
|
|
624 |
If f (x) = cos [π2] x + cos [-π2] x where [x] stands of the greatest integer function then a) f 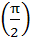 = −1 = −1 b) 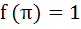 c) f (−π) = 0 d) f 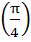 = 1 = 1
If f (x) = cos [π2] x + cos [-π2] x where [x] stands of the greatest integer function then a) f 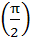 = −1 = −1 b) 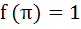 c) f (−π) = 0 d) f 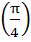 = 1 = 1
|
IIT 1991 |
03:36 min
|
|
625 |
Let p be a prime and m be a positive integer. By mathematical induction on m, or otherwise, prove that whenever r is an integer such that p does not divide r, p divides 
Let p be a prime and m be a positive integer. By mathematical induction on m, or otherwise, prove that whenever r is an integer such that p does not divide r, p divides 
|
IIT 1998 |
03:45 min
|